When discussing some of the side bets in blackjack, you’ll notice that I use terms like “expected return” and “house edge.” Here’s an explanation of those concepts.

Ken Uston - Fraud Or Pioneer?

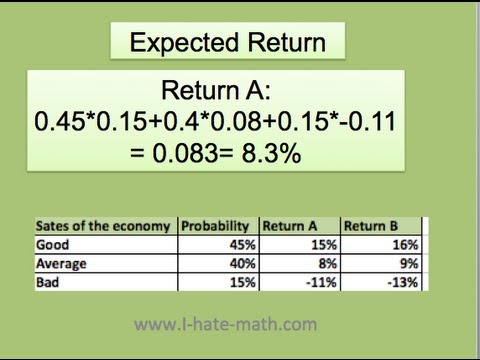
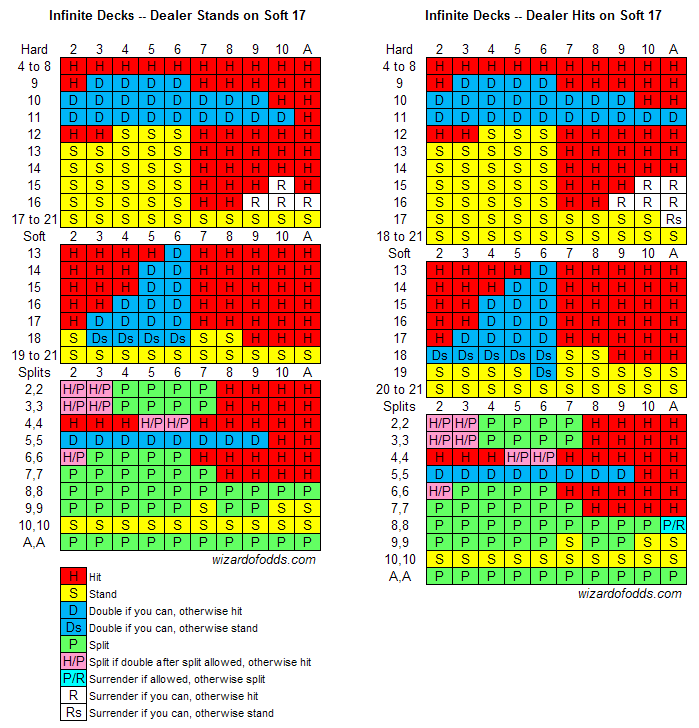
The expected value changes on a per hand basis and depends on more than one factor. I assume what you're referring to is the basic strategy for Blackjack having a negative EV in a typically available variant of Blackjack. Here's the first rub, the composition of remaining cards is. Twelve Blackjack calculators can be found below to answer various 'what if' questions. Win Rates and Standard Deviations used in these calculators can be found in the CVCX Online Viewer. Most of the methodologies used are either in or based on formulae in Don Schlesinger’s Blackjack Attack and page references are supplied below.

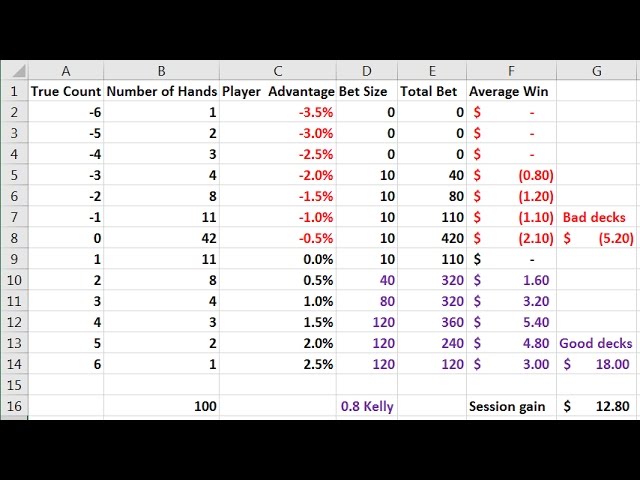
Blackjack Outcome Calculator Here, you can estimate the probability of winning or losing a specified amount of money given betsize, number of hands and advantage percentage. To find your advantage at Basic Strategy, link to the Blackjack Advantage Calculator. To use this calculator, fill in the four boxes below and press the Calculate button.

The expected return is the amount of money that you can expect to win or lose (in the long run) from each wager. For example, if you’re placing an even-money $1 bet, and your chances are 40% fora win and 60% for a loss. This would give you the following: $0.40 – $0.60 = -$0.20. The negative sign in front of the total means that for every dollar wagered you can expect to lose $0.20 (onaverage, over a long period of time—in the short run, anything can happen).

Here’s an example where you have multiple options for winning. Let’s say that you’re betting $1 on a slot machine, and you have a 20% chance of winning $4, a 25% chance of winning $3, and a 55%chance of losing. Here’s how the expected return would be calculated:

20% x $4 = $0.80

25% x $3 = $0.75

EV Per Hour - Blackjack And Card Counting Forums

55% x -$1 = -$0.55

$0.80 + $0.75 – $0.55 = $1

In this example, you could expect to win $1 per spin over the long term. Of course, no sane casino ever offers a slot machine game with a positive return.

The house edge, meanwhile, is the ratio of the average loss to the initial bet. Expressed as a percentage, this number demonstrates the subtle way in which casino games bleed aplayer dry.

For example, a 5% house edge means you can expect to lose $5 for every $100 wagered—or $0.05 for every $1. This might not sound like a lot, but it gives the casino a financial advantage over theplayer and allows for a steady accumulation of profit.

See Full List On Wizardofodds.com

Blackjack Expected Return Calculator Gross

Game designers and casinos carefully calculate the expected return and house edge before putting a game on the casino floor. The end result is that the house almost always has an advantage overthe player, which explains why the major casinos rake in obscene amounts of money on an annual basis.